Question

What is an equation of the line that passes through the point (2,−5) and is parallel to the line 6x+y=6

Answer 1

`y=-6x+7`

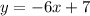
Explanation:

Hi there!

What we need to know:

• Linear equations are typically organized in slope-intercept form:
  `y=mx+b` where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis)
• Parallel lines always have the same slopes and different y-intercepts

1) Determine the slope (m)

`6x+y=6`

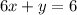
Rewrite this in slope-intercept form (to help us find the slope)

Subtract 6x from both sides

`6x+y-6x=-6x+6\\y=-6x+6`

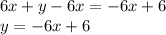
Now, we can identify clearly that the slope of this line is -6. Because parallel lines always have the same slopes, -6 will therefore be the slope of the line we're solving for. Plug this into
`y=mx+b`:

`y=-6x+b`

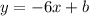
2) Determine the y-intercept (b)

`y=-6x+b`

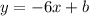
Plug in the given point (2,−5) and isolate b

`-5=-6(2)+b\\-5=-12+b`

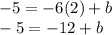
Add 12 to both sides

`-5+12=-12+b+12\\7=b`

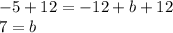
Therefore, the y-intercept is 7. Plug this back into
`y=-6x+b`:

`y=-6x+7`

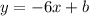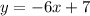
I hope this helps!