Question

The cost to fill a car’s tank with gas and get a car wash is a linear function of the capacity of the tank. The costs of a fill-up and a car wash for three different customers are shown in the table. Write an equation for the function in slope-intercept form. Then, find the cost of a fill-up and a car wash for a customer with a truck whose tank size is 26 gallons.

Tank size (gal)(x)
Total cost ($)f(x)
11
24.15
12
26.05
17
35.55

Answer 1

Explanation:

If this is linear, then the difference between each x/y coordinate is constant, representing the slope of a straight line. We can find the slope of that line by using the slope formula:

`m=(y_2-y_1)/(x_2-x_1)`

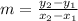
For use we will use the coordinates (11, 24.15) and (12, 26.05) from the data as our x and y values respectively. Filling in the formula:

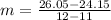`m=(26.05-24.15)/(12-11)` which gives us the cost per gallon of gas as

m = 1.90

Now that we know the cost per gallon of gas (which is also the slope of the line we are looking for), we will use that slope value, m, and write the equation in point-slope form and then solve it for y. Point-slope form of a line is

`y-y_1=m(x-x_1)` where y1 and x 1 are the coordinates from a given data point. We can use either one; regradless of the point we choose as x and y, we'll get the same exact answer every time. Let's use the coordinate (12, 26.05) and fill in the point-slope form of the line:

y - 26.05 = 1.90(x - 12) and then we will distribute and solve for y:

y - 26.05 = 1.90x - 22.80 and

y = 1.90x + 3.25

That's the equation we need to solve for what the second part of the question is asking us to, which is to find the cost, y, of filling a tank that is 26 gallons, x. Fill in 26 for x and solve for y:

y = 1.90(26) + 3.25 and

y = 49.40 + 3.25 so

y = $52.65