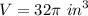Answer:
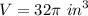
General Formulas and Concepts:
Symbols
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Geometry
Volume of a Cone Formula:
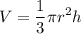
Explanation:
Step 1: Define
Identify
r = 4 in
h = 6 in
Step 2: Find Volume
- Substitute in variables [Volume of a Cone Formula]:
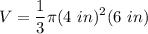
- Evaluate exponents:

- Multiply:
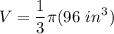
- Multiply: