Answer: Choice D
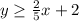
=======================================
Work Shown:

Note1: when dividing both sides by a negative number, the inequality sign flips.
----------------
Here's another approach that doesn't involve dividing both sides by a negative number.
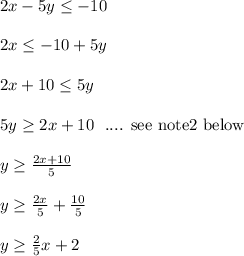
Note2: I swapped the left and right sides. Doing this swap means that the inequality sign must flip as well.