Question

Element X is a radioactive isotope such that every 13 years, its mass decreases by half. Given that the initial mass of a sample of Element X is 90 grams, how long would it be until the mass of the sample reached 62 grams, to the nearest tenth of a year?

Answer 1

The mass of Element X will reach 62 grams in 7.0 years.

Explanation:

We can find the time of decay of Element X by using the exponential decay equation:

`(dm)/(dt) = -\lambda t`

The solution of the above equation is:

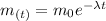`m_((t)) = m_(0)e^(-\lambda t)` (1)

Where:

t: is the time =?

λ: is the decay constant

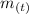`m_((t))`: is the mass at time t = 62 grams

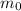`m_(0)`: is the initial mass = 90 grams

First, we need to calculate λ

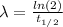`\lambda = (ln(2))/(t_(1/2))` (2)

Where
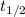`t_(1/2)` is the half-life = 13 years

By entering equation (2) into (1) and solving for "t" we have:

`(m_((t)))/(m_(0)) = e^{-(ln(2))/(t_(1/2))*t}`

`ln((m_((t)))/(m_(0))) = -(ln(2))/(t_(1/2))*t`

`t = -ln((m_((t)))/(m_(0)))((t_(1/2))/(ln(2))) = -ln((62)/(90))((13)/(ln(2))) = 7.0 y`

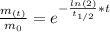
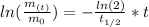
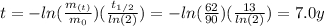
Therefore, the mass of Element X will reach 62 grams in 7.0 years.

I hope it helps you!