Given:
The compound inequality is:
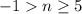
To find:
The reason for no solution of the given inequality.
Solution:
We have,
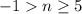
This inequality can be written as two separate inequalities.
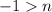 and
and
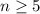
The inequality
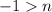 represents all real values less than -1.
represents all real values less than -1.
The inequality
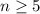 represents all real values greater than or equal to 5.
represents all real values greater than or equal to 5.
Both inequalities
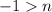 and
and
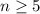 have no common values. Therefore, the given inequality has no solution. Hence proved.
have no common values. Therefore, the given inequality has no solution. Hence proved.