Answer:
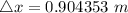
General Formulas and Concepts:
Physics
Energy
Gravitational Potential Energy Formula:
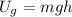
- m is mass (in kg)
- g is gravity (9.8 m/s²)
- h is height (in m)
Spring Potential Energy Formula:
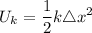
- k is spring constant (in N/m)
- Δx is displacement (in m)
Law of Conservation of Energy
Math
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Equality Properties
- Multiplication Property of Equality
- Division Property of Equality
- Addition Property of Equality
- Subtraction Property of Equality
Step-by-step explanation:
Step 1: Define
Identify
[Law of Conservation of Energy]
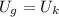 →
→
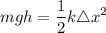
[Given] m = 45 kg
[Given] h = 15 m
[Given] k = 55000 n/m
[Solve] Δx
Step 2: Solve for Δx
- Substitute in variables [Law of Conservation of Energy]:
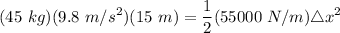
- Multiply:
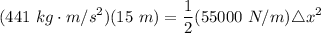
- Multiply:
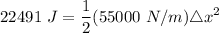
- Multiply:

- [Division Property of Equality] Divide 27500 N/m on both sides:

- [Equality Property] Square root both sides:
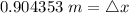
- Rewrite:
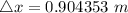
Topic: AP Physics 1 - Algebra Based
Unit: Energy
Book: College Physics