Question

The side lengths of a triangle are 5, 12, and 13. Is this a right triangle?

Answer 1

yes, it is right triangle

Explanation:

to know if it is right triangle or not, you need to assume that the longest side is the hypotenuse which is 13.

next, the angle between 12 and 5 must be 90 to prove that it was right triangle

to prove that, let's use cosinus theorem

`{13}^(2) = {12}^(2) + {5}^(2) - 2(12)(5) \cos(x) \\ 2(12)(5) \cos(x) = {12}^(2) + {5}^(2) - {13}^(2) \\ \cos(x) = \frac{ {12}^(2) + {5}^(2) - {13}^(2) }{2(12)(5)} \\ \cos(x ) = 0 \\ x = 90`

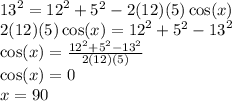
from that, we know it is a right triangle