Question

A survey of nonprofit organizations showed that online fundraising increased in the past year. Based on a random sample of 60 nonprofit organizations, the mean one-time gift donation in the past year was $75, with a standard deviation of $12. If you test the null hypothesis at the 0.05 level of significance, is there evidence that the mean one-time gift donation is greater than $70?

Answer 1

The p-value of the test is 0.001 < 0.05, which means that there is evidence at the 0.05 level of significance that the mean one-time gift donation is greater than $70.

Explanation:

Test if the mean one-time gift donation is greater than $70:

At the null hypothesis, we test if it is 70 or less, that is:

`H_0: \mu \leq 70`

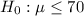
At the alternate hypothesis, we test if it is greater than 70, that is:

`H_1: \mu > 70`

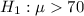
The test statistic is:

`t = (X - \mu)/((s)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis, s is the standard deviation of the sample and n is the size of the sample.

70 is tested at the null hypothesis:

This means that
`\mu = 70`

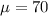
Based on a random sample of 60 nonprofit organizations, the mean one-time gift donation in the past year was $75, with a standard deviation of $12.

This means that
`n = 60, X = 75, s = 12`.

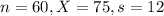Test statistic:

`t = (X - \mu)/((s)/(√(n)))`

`t = (75 - 70)/((12)/(√(60)))`

`t = 3.23`

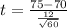
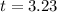
P-value of the test:

The p-value of the test is the probability of finding a sample mean above 75, which is a right-tailed test with t = 3.23 and 60 - 1 = 59 degrees of freedom.

Using a t-distribution calculator, this p-value is of 0.001.

The p-value of the test is 0.001 < 0.05, which means that there is evidence at the 0.05 level of significance that the mean one-time gift donation is greater than $70.