Answer:
The equation of curve is
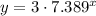 .
.
Explanation:
The exponential growth function is represented by the expression described below:
 (1)
(1)
Where:
 - Independent variable.
- Independent variable.
 - Dependent variable.
- Dependent variable.
 - Initial value of
- Initial value of
 .
.
 - Base.
- Base.
By deriving (1), we obtain an expression for the slope of the curve:
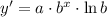 (2)
(2)
If we know that
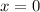 ,
,
 and
and
 , then we have the following system of equations:
, then we have the following system of equations:
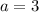 (3)
(3)
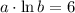 (4)
(4)
By (3) in (4):
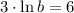

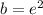
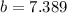
Therefore, the equation of curve is
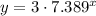 .
.