We are given with the equation x² + 10x - 39 = 0 and need to find x, so let's start ;
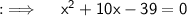
By using splitting the middle term method, Rewrite as ;
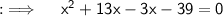
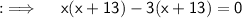
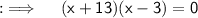
So, here either (x + 13) = 0 or (x - 3) = 0, when you equate both of them with 0, you will get x = -13 and x = 3
Hence, The required answer is -13 and 3