Answer:
The number of defective bulbs in the shipment should be around 15, give or take 4.
Explanation:
For each bulb, there are only two possible outcomes. Either it is defective, or it is not. The probability of a bulb being defective is independent of any other bulb. This means that the binomial probability distribution is used to solve this question.
Binomial probability distribution
Probability of exactly x successes on n repeated trials, with p probability.
The expected value of the binomial distribution is:
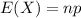
The standard deviation of the binomial distribution is:
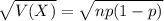
Suppose the probability at a light bulb factory of a bulb being defective is 0.11
This means that
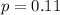
Shipment of 133 bulbs:
This means that
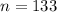
Mean and standard deviation:
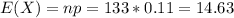
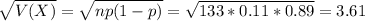
Rounding to the nearest integers:
The number of defective bulbs in the shipment should be around 15, give or take 4.