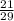Answer: The ratio represents the cosine of ∠H =
Explanation:
Given, In right triangle ΔHIJ, the measure of ∠J=90°, HJ = 21, JI = 20, and IH = 29.
To find: The ratio represents the cosine of ∠H.
According to trigonometry, the cosine of x =
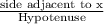
So cosine of
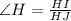
Cosine of
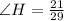
hence, the ratio represents the cosine of ∠H =