Question

A gas has a pressure of 6.5 atm at 540 K. What will the pressure be at 210 K if the volume does not change

Answer 1

The pressure at 210 K will be 2.53 atm.

Step-by-step explanation:

Gay-Lussac's law indicates that, as long as the volume of the container containing the gas is constant, as the temperature increases, the gas molecules move faster. Then the number of collisions with the walls increases, that is, the pressure increases. That is, the pressure of the gas is directly proportional to its temperature.

This law mathematically indicates that the quotient between pressure and temperature is constant:

`(P)/(T) =k`

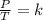
You want to study two different states, an initial state and a final state. You have a gas that is at a pressure P1 and at a temperature T1 at the beginning of the experiment. By varying the temperature to a new value T2, then the pressure will change to P2, and the following will be fulfilled:

`(P1)/(T1) =(P2)/(T2)`

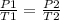
In this case:

• P1= 6.5 atm
• T1= 540 K
• P2= ?
• T2= 210 K

Replacing:

`(6.5 atm)/(540 K) =(P2)/(210 K)`

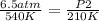
Solving:

`P2=210 K*(6.5 atm)/(540 K)`

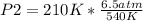
P2=2.53 atm

The pressure at 210 K will be 2.53 atm.