Answer:
x = 3, y = 7
or (3,7)
Explanation:
We are given the system of equations below:
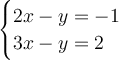
We are required to solve the system by substitution method. What we have to do is to isolate either x-term or y-term so we can use the method. I will be isolating y-term because it is faster due to having 1 as a coefficient.
By isolating y-term, just pick one of the given equations to isolate. No need to isolate the whole system. (I will be isolating y-term of the first equation.)
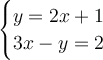
Then we substitute y = 2x+1 in the second equation.
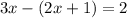
Use the distribution property.
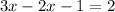
Isolate x-term to solve the equation.
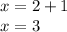
Since we are solving a system of equations. We have to solve for both x-value and y-value to complete. We have already found x-value, but nor y-value yet. Therefore, our next step is to substitute the value of x that we solved in any given equations. It's recommended to substitute in an equation that doesn't have high coefficient value. So I will be substituting x = 3 in the first equation.
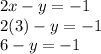
Isolate and solve for y-term.
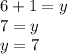
Since we substitute x = 3 and get y = 7. We can write in ordered pairs as (3,7)
Hence, the solution is (3,7)