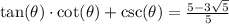Answer:
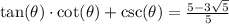
Explanation:
Given
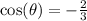
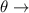 Quadrant III
Quadrant III
Required
Determine
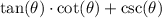
We have:
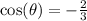
We know that:

This gives:
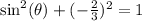
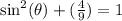
Collect like terms
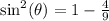
Take LCM and solve
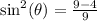
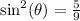
Take the square roots of both sides

Sin is negative in quadrant III. So:

Calculate
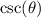
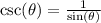
We have:

So:
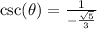
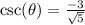
Rationalize
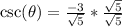
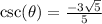
So, we have:
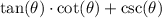
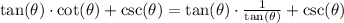
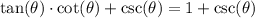
Substitute:
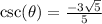
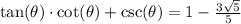
Take LCM