Answer:
Even positive
Explanation:
Given
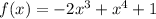
Required
The end behavior
We have:
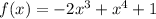
Rewrite as:

To describe the end behavior, we check the leading coefficient and the degree of the function.
We have:
Leading Coefficient = 1 (i.e. the coefficient of
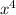 )
)
Degree = 4 (i.e. the power of
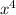 )
)
The degree of the function is even (i.e. 4)
The leading coefficient is positive (i.e. 1)
Hence, the end behavior is Even positive