Question

A certain town has 25,000 families. The average number of children per family is 2.6, with an SD of 0.80. The distribution is not normal, however, since 20% of the families have no children at all. (a) If one draws a simple random sample of five families from the 25,000, what is the chance that exactly two of the five sample families will have no children

Answer 1

0.2048 = 20.48% probability that exactly two of the five sample families will have no children.

Explanation:

For each children, there are only two possible outcomes. Either they have no children, or they do have children. The probability of a family not having children is independent of any other family. This means that the binomial probability distribution is used to solve this question.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

20% of the families have no children at all.

This means that
`p = 0.2`

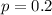
Five families:

This means that
`n = 5`

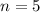
What is the chance that exactly two of the five sample families will have no children?

This is P(X = 2).

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 2) = C_(5,2).(0.2)^(2).(0.8)^(3) = 0.2048`

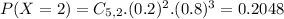
0.2048 = 20.48% probability that exactly two of the five sample families will have no children.