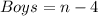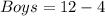Answer:
8 boys
Explanation:
Given
Let
 The initial number of boys
The initial number of boys
 Amount paid by each
Amount paid by each
Required
Number of boys that paid
After four boys left, the amount paid by each is:
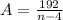
Before the four boys left, the amount each would have paid is:
 i.e. $8 less
i.e. $8 less
Make A the subject
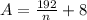
Equate both expressions
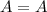
This gives:
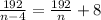
Take LCM
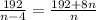
Cross multiply
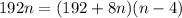
Open brackets
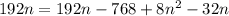
Subtract 192n from both sides
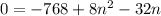
Rearrange
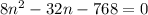
Divide through by 8
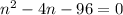
Expand
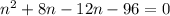
Factorize
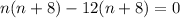
Factor out n + 8
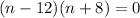
Split
 or
or
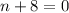
Solve for n
 or
or
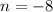
n cannot be negative
So:

The number of boys that stayed and paid are: