Question

Solve each of the following systems, if possible. indicate whether the system has a unique solution, infinitely many solutions, or no solution.

a. 2x + 10y = 9
3x + 15y = 13

b. 2x + 8y = 5
3x + 12y = 7.5

c. y = 3-x

d. 5x -8y = -1
-5/2 x + 4y = 1/2​

Answer 1

(a) No solution

(b) Infinitely many solution

(c) No solution

(d) Infinitely many solution

Explanation:

Solving (a):

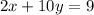`2x + 10y = 9` --- (1)

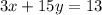`3x + 15y = 13` --- (2)

Multiply (1) by 1.5

1.5 * [
`2x + 10y = 9`]

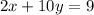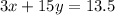`3x + 15y = 13.5` --- (3)

Subtract 2 and 3

`3x - 3x + 15y - 15y = 13.5 - 13`

`0 = 0.5`

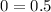
The above is false; Hence, the system has no solution

Solving (b):

`2x + 8y = 5` --- (1)

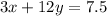`3x + 12y = 7.5` -- (2)

Multiply 1 by 1.5

1.5 * [
`2x + 8y = 5`]

`3x + 12y = 7.5`

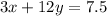
Subtract 2 and 3

`3x - 3x + 12y - 12y = 7.5 - 7.5`

`0 = 0`

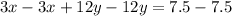
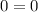
The above implies that the system has infinitely many solutions

Solving (c):

`y = 3 - x`

A second equation is required to solve for (c)

Solving (d):

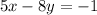`5x - 8y = -1` --- (1)

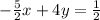`-(5)/(2)x+ 4y = (1)/(2)` --- (2)

Multiply (2) by 2

`-5x + 8y = 1` --- (3)

Add 1 and 3

`5x - 5x -8y + 8y = -1 + 1`

`0 = 0`

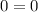
The above implies that the system has infinitely many solutions