Question

Which expressions correctly represent the area of the triangle XYZ?

Answer 1

Options (2) and Option (1)

Explanation:

Area of the ΔXYZ =
`(1)/(2)(\text{Base})(\text{Height})`

=
`(1)/(2)(XZ)h`

=
`(1)/(2)(18)h`

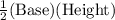
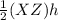
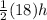
By applying sine rule in the ΔYPZ,

sin(38°) =
`\frac{\text{Opposite side}}{\text{Hypotenuse}}`

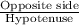
sin(38°) =
`(h)/(14)`

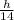
h = 14[sin(38°)]

Therefore, area of the given triangle =
`(1)/(2)(18)(14)sin(38)`

By applying sine rule in ΔYPZ,

sin(58°) =
`\frac{\text{Opposite side}}{\text{Hypotenuse}}`

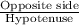
sin(58°) =
`(h)/(10)`

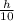
h = 10sin(58°)

Area of ΔXYZ =
`(1)/(2)(18)(10)sin(58)`

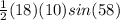
Therefore, Options (2) and Option (1) are the correct options.