Question

The National Collegiate Athletic Association (NCAA) requires colleges to report the graduation rates of their athletes. At one large university, 91% of all students who started their studies in 2000-2010 graduated within 6 years. A sports reporter contacted 152 athletes randomly sampled from that same university and time period and found that 132 of them had graduated within 6 years.

(a) (5 points) Perform the appropriate hypothesis test to determine whether this is significant evidence that the percentage of athletes who graduate is less than for the student population at large, using the significance level a = 0.05. Remember for state the null and alternative hypotheses, the decision rule, and your conclusion.
(b) (3 points) Calculate the P-value for this test. Explain how this P-value can be use to test the hypotheses in part (a).

Answer 1

a)

The null hypothesis is
`H_0: p = 0.91`.

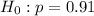The alternate hypothesis is
`H_1: p < 0.91`.

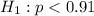The decision rule is: accept the null hypothesis for
`z > -1.645`, reject the null hypothesis for
`z < -1.645`.

Since
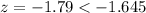`z = -1.79 < -1.645`, we reject the null hypothesis and accept the alternate hypothesis that that percentage of athletes who graduate is less than for the student population at large.

b)

The p-value for this test is 0.0367. Since this p-value is less than the significance level of
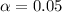`\alpha = 0.05`, we reject the null hypothesis and accept the alternate hypothesis that that percentage of athletes who graduate is less than for the student population at large.

Explanation:

Question a:

Perform the appropriate hypothesis test to determine whether this is significant evidence that the percentage of athletes who graduate is less than for the student population at large:

At the null hypothesis, we test if the proportion is the same as the student population, of 91%. Thus:

`H_0: p = 0.91`

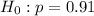
At the alternate hypothesis, we test that the proportion for athletes is less than 91%, that is:

`H_1: p < 0.91`

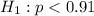
The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

Test if the proportion is less at the 0.05 level:

The critical value is z with a p-value of 0.05, that is, z = -1.645. Thus, the decision rule is: accept the null hypothesis for
`z > -1.645`, reject the null hypothesis for
`z < -1.645`.

0.91 is tested at the null hypothesis:

This means that
`\mu = 0.91, \sigma = √(0.91*0.09)`

A sports reporter contacted 152 athletes randomly sampled from that same university and time period and found that 132 of them had graduated within 6 years.

This means that
`n = 152, X = (132)/(152) = 0.8684`

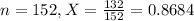
Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (0.8684 - 0.91)/((√(0.91*0.09))/(√(152)))`

`z = -1.79`

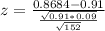
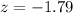
Since
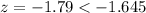`z = -1.79 < -1.645`, we reject the null hypothesis and accept the alternate hypothesis that that percentage of athletes who graduate is less than for the student population at large.

(b) (3 points) Calculate the P-value for this test. Explain how this P-value can be use to test the hypotheses in part (a).

The p-value of the test is the probability of finding a sample proportion of 0.8684 or below. This is the p-value of z = -1.79.

Looking a the z-table, z = -1.79 has a p-value of 0.0367.

The p-value for this test is 0.0367. Since this p-value is less than the significance level of
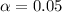`\alpha = 0.05`, we reject the null hypothesis and accept the alternate hypothesis that that percentage of athletes who graduate is less than for the student population at large.