Question

Let measure of angle B be 18 degrees and BC = 9. Find the length of AC, BA and the measure of angle C. Round to 1 decimal place when needed. Show all of your work.

Answer 1

m∠C = 72°

BA = 8.6

AC = 2.8

Explanation:

We can start by finding the sum of all angles in a triangle to find angle C. The sum of all angles is 180°, so we can write the equation:

m∠A + m∠B + m∠C = 180°

And substitute what we have:

90° + 18° + m∠C = 180°

108° + m∠C = 180°

m∠C = 72°

In order to find the side BA, we can use trig functions. We are given the hypotenuse and we are looking for the side adjacent to ∠B. The trig function that relates the two is cosine. We can set up the equation as follows:

cos(∠B) =
`(BA)/(BC)`

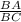
cos(18°) =
`(BA)/(9)`

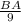
9 * cos(18°) = BA

BA ≈ 8.559508647

And when we round, we get:

BA = 8.6

In order to find the side AC, we can use trig functions. We are given the hypotenuse and we are looking for the side opposite to ∠B. The trig function that relates the two is sine. We can set up the equation as follows:

sin(∠B) =
`(AC)/(BC)`

sin(18°) =
`(AC)/(9)`

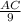
9 * sin(18°) = AC

AC ≈ 2.781152949

We can round this to:

AC = 2.8