Question

PLSSSSS HELP I DONT GET THIS PROBLEMMMM

...Using the above data table and graph, calculate the total energy in Joules required to raise the temperature of 15.0g of ice at -5.00ºC to steam at 35.0ºC

a) 7730 J
b) 7740 J
c) 7370 J
d) 7340 J

Answer 1

C. 7370 joules.

Step-by-step explanation:

There is a mistake in the statement. Correct form is described below:

Using the above data table and graph, calculate the total energy in Joules required to raise the temperature of 15 grams of ice at -5.00 °C to water at 35 °C.

The total energy needed to raise the temperature is the combination of latent and sensible heats, all measured in joules, and represented by the following model:

`Q = m\cdot [c_(i) \cdot (T_(2)-T_(1))+L_(f) + c_(w)\cdot (T_(3)-T_(2))]` (1)

Where:

`m` - Mass of the sample, in grams.

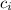`c_(i)` - Specific heat of ice, in joules per gram-degree Celsius.

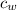`c_(w)` - Specific heat of water, in joules per gram-degree Celsius.

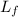`L_(f)` - Latent heat of fusion, in joules per gram.

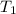`T_(1)` - Initial temperature of the sample, in degrees Celsius.

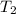`T_(2)` - Melting point of water, in degrees Celsius.

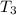`T_(3)` - Final temperature of water, in degrees Celsius.

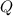`Q` - Total energy, in joules.

If we know that
`m = 15\,g`,
`c_(i) = 2.06\,(J)/(g\cdot ^(\circ)C)`,
`c_(w) = 4.184\,(J)/(g\cdot ^(\circ)C)`,
`L_(f) = 334.72\,(J)/(g)`,
`T_(1) = -5\,^(\circ)C`,
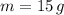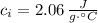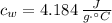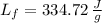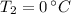`T_(2) = 0\,^(\circ)C` and
`T_(3) = 35\,^(\circ)C`, then the final energy to raise the temperature of the sample is:

`Q = (15\,g)\cdot \left[\left(2.06\,(J)/(g\cdot ^(\circ)C) \right)\cdot (5\,^(\circ)C)+ 334.72\,(J)/(g) + \left(4.184\,(J)/(g\cdot ^(\circ)C)\right)\cdot (35\,^(\circ)C) \right]`

`Q = 7371.9\,J`

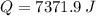
Hence, the correct answer is C.