Question

The plant-breeding department at a major university developed a new hybrid boysenberry plant called Stumptown Berry. Based on research data, the claim is made that from the time shoots are planted 90 days on average are required to obtain the first berry. A corporation that is interested in marketing the product tests 60 shoots by planting them and recording the number of days before each plant produces its first berry. The sample mean is 92.3 days. The corporation wants to know if the mean number of days is different from the 90 days claimed. What are the correct hypotheses?

a) H0:μ≠90
H1:μ=90
b) H0:μ=90
H1:μ≠90
c) H0:μ=92.3
H1:μ≠92.3
d) H0:p=92.3%
H1:p≠92.3%
e) H0:p=90%
H1:p≠90%

Answer 1

b) H0:μ=90, H1:μ≠90

Explanation:

The corporation wants to know if the mean number of days is different from the 90 days claimed.

This means that at the null hypothesis, we test if the mean number is the claimed value of 90, and so:

`H_0: \mu = 90`

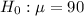
The corporations wants to know if the mean number is different, and thus, at the alternate hypothesis, we test if the mean number is different from 90, that is:

`H_1: \mu \\eq 90`

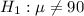
This means that the correct answer is given by option b.