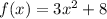Answer:
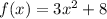
Explanation:
We are given the first derivative of
 and the value of
and the value of
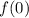 .
.
To go from the first derivative to the original function, we integrate.
Therefore:
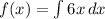
To integrate, we add 1 to the power and divide by the new power:
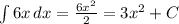
Because we have an indefinite integral, we have to add the constant,
 , to the end.
, to the end.
So:
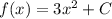
We know
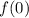 so we can find the constant
so we can find the constant
 .
.
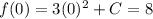

Therefore