Answer:
Common difference is 6
Possible values of n is n > 8
Explanation:
(i) The nth term (Uₙ) of an arithmetic series is given by
Uₙ = a + (n-1)d --------------------(i)
and the sum of the series is given by;
Sₙ =
 (2a + (n-1)d) ---------------------(ii)
(2a + (n-1)d) ---------------------(ii)
Where;
a = first term of the series
d = common difference of the series
n = the term or number of terms to be added.
Interpret the first statement
The third term of the series is 70. i.e
n = 3
Uₙ = U₃ = 30
Substitute these values into equation (i) as follows;
=> 30 = a + (3 - 1)d
=> 30 = a + 2d
=> a = 30 - 2d --------------------(iii)
Interpret the next statement
the sum of the first 10 terms of the series is 450. i.e
n = 10
Sₙ = S₁₀ = 450
Substitute these values into equation (ii) as follows:
=> 450 =
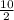 (2a + (10-1)d)
(2a + (10-1)d)
=> 450 = 5(2a + 9d)
Divide both sides by 5
=> 90 = 2a + 9d ---------------------------(iv)
Solve equations (iii) and (iv) simultaneously
Substitute the value of a in equation(iii) into equation (iv) and solve as follows
=> 90 = 2(30 - 2d) + 9d
=> 90 = 60 - 4d + 9d
=> 90 = 60 + 5d
=> 5d = 90 - 60
=> 5d = 30
Divide both sides by 5
=> d = 6
Therefore, the common difference, d is 6
(ii) Given
Sₙ > 350
From equation (ii), this implies that,
 (2a + (n-1)d) > 350 -------------------(v)
(2a + (n-1)d) > 350 -------------------(v)
Let's get the value of the first term 'a'
Substitute the value of d = 6 into equation (iii) and solve as follows
=> a = 30 - 2(6)
=> a = 30 - 12
=> a = 18
Substitute the values of a and d into inequality (v) and solve
 (2(18) + (n-1)6) > 350
(2(18) + (n-1)6) > 350
 (36 + 6n - 6) > 350
(36 + 6n - 6) > 350
 (36 - 6 + 6n) > 350
(36 - 6 + 6n) > 350
 (30 + 6n) > 350
(30 + 6n) > 350
Multiply both sides by 2
n(30 + 6n) > 700
30n + 6n² > 700
6n² + 30n > 700
Divide through by 2
3n² + 15n > 350
3n(n+5) > 350
Solve the quadratic inequality
Since n specifies the number of terms to be added, it will always be a whole number. Therefore, using trial and error method, the values of n that will satisfy the above inequality will be values greater than 8. i.e
n > 8
In other words, for all values of n greater than 8, the above inequality will be satisfied.