Answer:
A. Adding the values of the intercepted arcs and that is equal to twice the angle measure
Explanation:
The intersecting chords theorem states that when four line segments are formed by two chords intersecting in a circle, the product of the two line segments on one chord is equal to the product of the two line segments on the other chord
The angles of intersecting chords theorem states that the angles formed by the intersecting chords one half the sum of the arcs intercepted by the chords
In the diagram attached, we have;
∠x = ∠y + ∠z
m
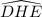 = 2·∠z, m
= 2·∠z, m
 = 2·∠y
= 2·∠y
∴ ∠x = (1/2) × m
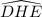 + m
+ m

m
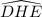 + m
+ m
 = 2 × ∠x
= 2 × ∠x
The correct option is therefore adding the values of the intercepted arcs and that is equal to twice the angle measure.