Question

Sir Percival and his horse are standing still on a field of honor when Sir Rodney, atop his steed gallops by with a constant velocity of 3.00m/s. He flips Percival off as he passes by! Percival instantly begins to give chase with an acceleration of 0.600m/s2. Using Percival’s at rest position as zero, determine the time it takes for Percival to catch up to Rodney.

a. 4.0 s
b. 6.0 s
c. 8.0 s
d. none of these

Answer 1

d. none of these

Step-by-step explanation:

From the given information:

Let assume that Percival catches Sir Rodney's horse in time "t" after covering a certain distance "s"

Then, using the second equation of motion:

`S = ut + (1)/(2)at^2`

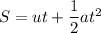
FOR Percival, we have:

`S = (0* t ) + (1)/(2) * 0.6 \ m/s * t^2 \\ \\ S = 0.3 \ m/s * t^2 -- -- (1)`

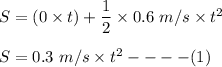
FOR Sir Rodney;

`S = ut + (1)/(2)at^2`

`S = (3* t ) + (1)/(2) * 0 * t^2 \\ \\ S =3t--- (2)`

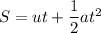
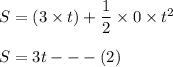
Equating both equations together; we have:

0.3t² = 3t

0.3t² - 3t = 0

0.3t(t - 10) = 0

If Percival's position at rest = 0

Then; t = 10 s.