Answer:
d. 89°
Explanation:
The given measure of the angles formed are;
m∠AED = 48°, m
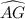 = 175°
= 175°
According to circle theorem, the angle formed by a chord and a tangent of a circle is given by half of the measure of the arc intercepted by the chord in the direction of the angle;
Therefore;
m∠AED = (1/2) × m
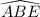 = 48°
= 48°
∴ m
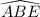 = 2 × 48° = 96°
= 2 × 48° = 96°
m∠AEF = (1/2) × m
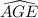
m
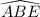 + m
+ m
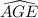 = 360° (angle round a circle)
= 360° (angle round a circle)
∴ m
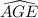 = 360° - m
= 360° - m
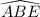 = 360° - 96° = 264°
= 360° - 96° = 264°
m
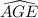 = m
= m
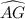 + m
+ m
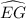
∴ m
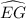 = m
= m
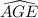 - m
- m
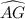 = 264° - 175° = 89°
= 264° - 175° = 89°
m
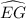 = 89°.
= 89°.