Question

WHAT IS THE LENGTH OF AC? ROUND TO ONE DECIMAL PLACE. DONT HAVE TO SHOW WORK​ helpppp

Answer 1

AC ≈ 5.5

Explanation:

Angle bisector theorem states that "angle bisector of an angle divides the opposite side into two segments which are proportional to the other two sides of the triangle."

By applying this theorem in the given ΔABC,

AD is the angle bisector of ∠CAB which divides the opposite side of the angle into two segments BD and CD.

Therefore,
`(AB)/(BD)=(AC)/(CD)`

`(5.1)/(4)=(AC)/(4.3)`

`AC=(5.1* 4.3)/(4)`

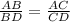
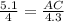
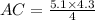
AC = 5.4825

AC ≈ 5.5 units