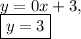Answer:
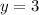
Explanation:
The slope of a line is given as
 , where
, where
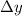 is change in y-value and
is change in y-value and
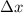 is change in x-value for any two points the line passes through.
is change in x-value for any two points the line passes through.
Using the points (0, 3) and (4, 3) as given in the problem, we have:
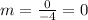 . Therefore, the slope of this line is 0.
. Therefore, the slope of this line is 0.
In slope-intercept form, we have
 . Since we've found out that
. Since we've found out that
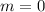 the entire term
the entire term
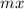 will be equal to 0 and we are left with
will be equal to 0 and we are left with
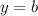 , where
, where
 is the y-intercept. We can plug in the coordinates of any point the line passes through to find this value:
is the y-intercept. We can plug in the coordinates of any point the line passes through to find this value:
Using the point (4, 3) as given in the problem:
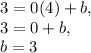
Thus, the equation of our line is