Answer:
a) R = 172.82 m, 5.3 north of east b) v = (0.24 ± 0.01) m / s
Step-by-step explanation:
a) the displacement is a vector, so the easiest method to lock is looking for each component
let's start decomposing the vectors
x₁ = - 160 m
second shift
angle 45 south of east
cos (-45) = x₂ / d₂
sin (-45) = y₂ / d₂
x₂ = d₂ cos 45
y₂ = -d₂ sin 45
x₂ = 180 cos 45 = 127.28 m
y₂ = -180 sin 45 = - 127.28 m
third shift
cos 35 = x₃ / d₃
sin 35 = y₃ / d₃
x₃ = d₃ cos 35
y₃ = d₃ sin 35
x₃ = 250 cos 35 = 204.79 m
y₃ = 250 sin 35 = 143.39 m ₃
X axis
x_total = x₁ + x₂ + x₃
x_total = -160 +127.28 +204.79
x_total = 172.07 m
Y axis
y_total = y₁ + y₂ + y₃
y_total = 0 - 127.28 + 143.39
y_total = 16.11 m
to compose the displacement we use the Pythagorean theorem
R =
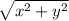
R =
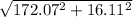
R = 172.82 m
in angle is
tan θ = y_total / x_total
ten θ = 16.11 / 172.07 = 0.0936
θ = tan⁻¹ 0.0936
θ = 5.3
angle is 5.3 north of east
b) the error in the distance is 5%,
e% = ΔR/R 100
ΔR = e% R / 100
ΔR = 5 172.82 / 100
ΔR = 8.6 m
the time error Dt = 20 s
We calculate the speed and this we calculate the error
v = R / t
v = 172.82 / 12 60
v = 0.240027 m / s
the error in this magnitude is
Δv =
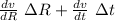
Δv =
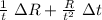
Δv =
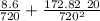
Δv = 0.013 m / s
the measurement result is
v = (0.24 ± 0.01) m / s