Answer:

Explanation:
Since the base is twice as long as the height, set up the following proportion:
 .
.
The area of the a right triangle is equal to
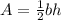 , where
, where
 is a base of the triangle and
is a base of the triangle and
 is the respective height.
is the respective height.
To solve for height, set up the equation (substituing
 :
:
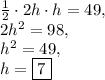
To solve for base, substitute
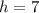 in the proportion
in the proportion
 :
:
