Given:
An exponential function goes through points (0,12) and (3,6144).
To find:
The exponential function.
Solution:
The general exponential function is:
 ...(i)
...(i)
The function goes through point (0,12). Substituting
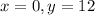 , we get
, we get
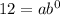
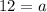
The function goes through point (3,6144). Substituting
 in the general exponential function, we get
in the general exponential function, we get
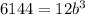
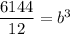
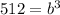
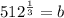
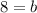
Putting
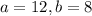 in (i), we get
in (i), we get
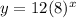
Therefore, the required exponential function is
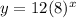 .
.