Question

The average teaching salary in Georgia is $48,553 per year. The school districts in the metro Atlanta area boast that they pay more. A company that is running a career fair decides to take a random sample of 228 teachers from the metro Atlanta area and record their salaries. The sample mean is $49,021, with a standard deviation of $3,127.

Required:
Do the data provide statistically significant evidence at the 0.06 kevel that the average teaching stay in the metro Atlanta area is greater than the state average?

Answer 1

it's D

Explanation:

Answer 2

The p-value of the test is 0.0119 < 0.06, which means that the data provides statistically significant evidence at the 0.06 level that the average teaching stay in the metro Atlanta area is greater than the state average.

Explanation:

The average teaching salary in Georgia is $48,553 per year. The school districts in the metro Atlanta area boast that they pay more.

At the null hypothesis, we test if they pay the average, that is:

`H_0: \mu = 48553`

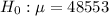
At the alternate hypothesis, we test if they pay more, that is:

`H_1: \mu > 48553`

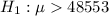
The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

48553 is tested at the null hypothesis:

This means that
`\mu = 48553`

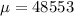
Sample of 228 teachers from the metro Atlanta area and record their salaries. The sample mean is $49,021, with a standard deviation of $3,127.

This means that
`n = 228, X = 49021, \sigma = 3127`

Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (49021 - 48553)/((3127)/(√(228)))`

`z = 2.26`

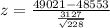
P-value of the test and decision:

The p-value of the test is the probability of finding a sample mean above 49021, which is 1 subtracted by the p-value of z = 2.26.

Looking at the z-table, z = 2.26 has a p-value of 0.9881

1 - 0.9881 = 0.0119

The p-value of the test is 0.0119 < 0.06, which means that the data provides statistically significant evidence at the 0.06 level that the average teaching stay in the metro Atlanta area is greater than the state average.