Answer:

Step-by-step explanation:
If you recall the unit circle [from the polar graph], you would have no trouble at all figuring this out, but sinse you have trouble, do not worry about it. So, here is what you should have realised:
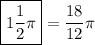
If you did not notise,
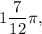 or
or
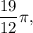 is just
is just
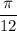 more than
more than
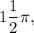 which means the exact value will either be a radical, followed by an integer or just a radical. In this case, accourding to the unit circle, you will have a radical, followed by an integer, and that will be this:
which means the exact value will either be a radical, followed by an integer or just a radical. In this case, accourding to the unit circle, you will have a radical, followed by an integer, and that will be this:
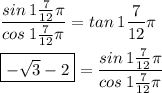
It is all about memorisation of the unit circle, which I know is difficult, but you will get used to it soon.
*Now, if you had to find
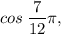 then the exact value would be the OPPOCITE of the exact value of
then the exact value would be the OPPOCITE of the exact value of
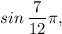 which is
which is
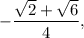 because you would be crossing into the second quadrant where the x-coordinates are negative, accourding to both the cartesian and polar graphs. In addition, sinse you ALREADY have both values for sine and cosine [accourding to your PREVIOUS QUESTION ASKED], all you need to do is divide both values in the order given above to arrive at the exact value for
because you would be crossing into the second quadrant where the x-coordinates are negative, accourding to both the cartesian and polar graphs. In addition, sinse you ALREADY have both values for sine and cosine [accourding to your PREVIOUS QUESTION ASKED], all you need to do is divide both values in the order given above to arrive at the exact value for
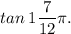
I am joyous to assist you at any time.