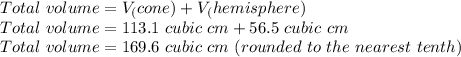Answer:
The total volume of the ice cream, when the cone is completely filled and a hemisphere is added on top, is approximately
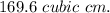
Explanation:
To find the total volume of the ice cream, we need to calculate the volume of the cone and the volume of the hemisphere separately, and then add them together.
First, let's find the volume of the cone. The formula for the volume of a cone is
 , where
, where
 is the volume,
is the volume,
 is the radius, and
is the radius, and
 is the height.
is the height.
Given that the cone has a diameter of
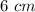 , we can find the radius by dividing the diameter by 2. So, the radius
, we can find the radius by dividing the diameter by 2. So, the radius
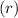 of the cone is
of the cone is
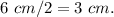
The height
 of the cone is given as
of the cone is given as
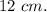
Now, we can substitute the values into the formula and calculate the volume of the cone:
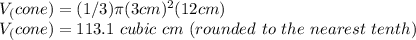
Next, let's find the volume of the hemisphere. The formula for the volume of a hemisphere is
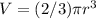 , where
, where
 is the volume and
is the volume and
 is the radius.
is the radius.
Since the radius of the cone and the hemisphere is the same
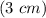 , we can use the same value.
, we can use the same value.
Now, we can substitute the value into the formula and calculate the volume of the hemisphere:

Finally, we add the volume of the cone and the volume of the hemisphere together to find the total volume of the ice cream: