Answer:
8.5 ft
Explanation:
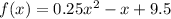
To find the minimum point of the function, differentiate:
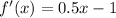
set to zero and solve for x:

Substitute found value of x into function to find y (height):

Therefore, the lowest point on the bottom edge of the banner is 8.5 ft above the floor.