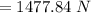Answer:
The correct answer will be "1477.84 N".
Step-by-step explanation:
Given that,
Mass,
m = 1.6 mg
or,
= 1600 kg
Initial velocity,
u = 72 km/h
=
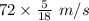
=
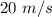
Final velocity,
v = 18 km/h
=

=

Covered distance,
s = 250 m
By using the below relation, we get
⇒
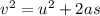
On putting the values, we get
⇒
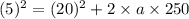
⇒
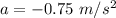 (shows the deceleration)
(shows the deceleration)
Slope will be given as 1 in 25, then
⇒
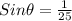
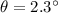
hence,
As we know,
⇒
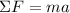
or,
⇒
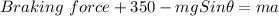
⇒
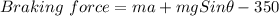
On substituting all the values, we get
⇒
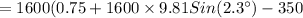
⇒