Answer:
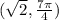 or
or
 (using the angle in the radian form)
(using the angle in the radian form)
Explanation:
Here, we want to write the given rectangular coordinate in its polar form
Mathematically, we start by identifying the quadrant in which we have the point
The point is located in the the fourth quadrant
We have the general polar coordinate form as;
(r,θ)
where;
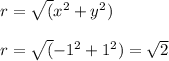
We have the theta value calculated as;
theta = arc tan (y/x)
y = -1 and x = 1
theta = arc tan (-1/1)
theta = arc tan (-1)
theta = 45+270 = 315
So, we have the polar coordinate expression as;
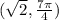
or in radians as;
