Question

Find the distance between the two points rounding to the nearest tenth (if necessary).

(-6, 6) and (-3,3)

Answer 1

`\boxed {\boxed {\sf d\approx 4.2}}`

Explanation:

The formula for distance is:

`d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2`

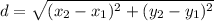
Where (x₁, y) and (x₂, y₂) are the points.

We are given (-6, 6) and (-3, 3). If we match the value and its corresponding variable, we see that:

• x₁= -6
• y₁ = 6
• x₂ = -3
• y₂ = 3

Substitute the values into the formula.

`d= \sqrt{ (-3 - -6)^2+(3-6)^2`

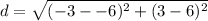
Solve inside the parentheses.

• -3 --6 = -3+6 = 3
• 3-6 = -3

`d= \sqrt{(3)^2+ (-3)^2`

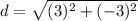
Solve the exponents.

• (3)²= 3*3= 9
• (-3)²= -3*-3 =9

`d= \sqrt{9+9`

Add.

`d= \sqrt18`

`d= 4.24264069`

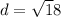
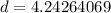
Round to the nearest tenth. The 4 in the hundredth place tells us to leave the 2 in the tenth place.

`d \approx 4.2`

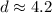
The distance between the two points is apprximately 4.2