Answer:
No. Remember, a right angle must have a 90 degree angle. We can find the lengths with the Pythagorean Theorem.
Explanation:
Given the length 7, 10, and 12, we can assume that 12 is the hypotenuse (it is the longest length).
- we can use 7 and 10 interchangeably.
Fill in the equation,
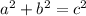
where c = 12, and a or b = 7 or 10.
To indicate if the given lengths would form a right angle, we can only input 7 or 10, not both.
Therefore,
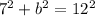 or
or
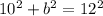
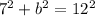 ==> 49 + b^2 = 144 ==> b=
==> 49 + b^2 = 144 ==> b=
 ==> 9.746
==> 9.746
b= 9.7, not 10.
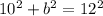 ==> 100 + b^2 = 144 ==> b =
==> 100 + b^2 = 144 ==> b =
 ==> 6.633
==> 6.633
b= 6.6, not 7.
Therefore, the lengths 7, 10, and 12, does NOT make a right triangle.
Hope this helps!