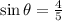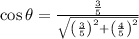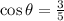Answer:
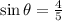 ,
,
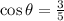
Explanation:
Let be
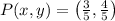 the end of the terminal side of angle
the end of the terminal side of angle
 in standard position, that is, an angle measured with respect to +x semiaxis. By Trigonometry, we know that the sine and the cosine of the angle are, respectively:
in standard position, that is, an angle measured with respect to +x semiaxis. By Trigonometry, we know that the sine and the cosine of the angle are, respectively:
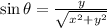 (1)
(1)
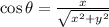 (2)
(2)
If we know that
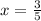 and
and
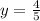 , then the sine and the cosine of the angle are:
, then the sine and the cosine of the angle are: