Answer:
Explanation:
To get the solution lying in the shaded region,
Justify the equation with the given options,
Option (1)
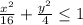
For (1, 1),
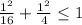
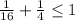

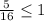
True.
Therefore, point (1, 1) justifies the equation.
Option (2)
For (4, 1),
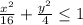

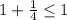

False.
Therefore, point (4, 1) doesn't justify the equation.
Option (3)
For (-1, 2),
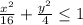
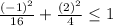
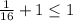

False.
Therefore, point (-1, 2) doesn't justify the equation.
Option (4)
For (2, -2),
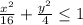
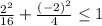
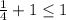

False.
Therefore, point (2, -2) doesn't justify the equation.
Option (1) will be the answer.