Answer:
V= 76 π cubic units
Explanation:
y=
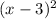 ----->
----->
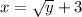
Here we will apply the risk method.
Risk method: consider a strip of length x and width dy as
a) Radius= R(y) = x =
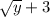
[We rotate this disc about y-axis to get a disk]
b) Cross-section area = A(y)= π
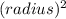
= A(y)= π (y+9+6
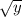 )
)
--------> Volume of the disc = A(y) x dy
c) Required volume= v =
 A(y) x d(y)
A(y) x d(y)
V=
 π (y + 9 + 6
π (y + 9 + 6
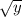 ) dy
) dy
V= π [
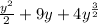
![]_(0) ^(4)](https://img.qammunity.org/2022/formulas/mathematics/college/mivu6zjch4xb4a7oiyv88cy3wakb096bf8.png)
V= 76 π cubic units.