Question

A publisher reports that 75% of their readers own a particular make of car. A marketing executive wants to test the claim that the percentage is actually different from the reported percentage. A random sample of 280 found that 70% of the readers owned a particular make of car. Is there sufficient evidence at the 0.02 level to support the executive's claim?

Answer 1

The p-value of the test is of 0.0536 > 0.02, which means that there is not sufficient evidence at the 0.02 level to support the executive's claim.

Explanation:

A publisher reports that 75% of their readers own a particular make of car. A marketing executive wants to test the claim that the percentage is actually different from the reported percentage.

At the null hypothesis, we test if the proportion is of 75%, that is:

`H_0: p = 0.75`

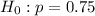
At the alternate hypothesis, we test if the proportion is different of 75%, that is:

`H_1: p \\eq 0.75`

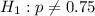
The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

75% is tested at the null hypothesis:

This means that
`\mu = 0.75, \sigma = √(0.75*0.25)`

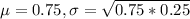
A random sample of 280 found that 70% of the readers owned a particular make of car.

This means that
`n = 280, X = 0.7`

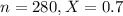
Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (0.7 - 0.75)/((√(0.75*0.25))/(√(280)))`

`z = -1.93`

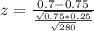
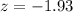
P-value of the test and decision:

The p-value of the test is the probability of finding a sample proportion differing from 0.75 by at least |0.7 - 0.75| = 0.05, which is P(|z| > 1.93), which is 2 multiplied by the p-value of z = -1.93.

Looking at the z-table, z = -1.93 has a p-value of 0.0268.

2*0.0268 = 0.0536.

The p-value of the test is of 0.0536 > 0.02, which means that there is not sufficient evidence at the 0.02 level to support the executive's claim.