Question

Kryptonite is a material found on the planet Krypton and has various effects, most importantly on Superman. The most common types are green kryptonite and red kryptonite, but it comes in a variety of colors. All varieties of kryptonite satisfy the modified separable kryptonite decay equation

dy/dt = y(1/t- k) for t > 0

where y is the amount of kryptonite present t hours after we begin to measure the decay of the kryptonite. After one hour, 15 grams of red kryptonite are present, but amazingly enough, after three hours, 30 grams of red kryptonite are present. Strange stuff, indeed! What is the maximum amount of red kryptonite present, and after how much time will this occur?

Answer 1

The maximum amount of red kryptonite present is 33.27 g after 4.93 hours.

Explanation:

dy/dt = y(1/t - k)

separating the variables, we have

dy/y = (1/t - k)dt

dy/y = dt/t - kdt

integrating both sides, we have

∫dy/y = ∫dt/t - ∫kdt

㏑y = ㏑t - kt + C

㏑y - ㏑t = -kt + C

㏑(y/t) = -kt + C

taking exponents of both sides, we have

`(y)/(t) = e^(-kt + C) \\(y)/(t) = e^(-kt)e^(C) \\(y)/(t) = Ae^(-kt) (A = e^(C))\\y = Ate^(-kt)`

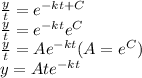
when t = 1 hour, y = 15 grams. So,

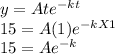`y = Ate^(-kt)\\15 = A(1)e^(-kX1)\\15 = Ae^(-k)`(1)

when t = 3 hours, y = 30 grams. So,

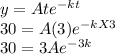`y = Ate^(-kt)\\30 = A(3)e^(-kX3)\\30 = 3Ae^(-3k)` (2)

dividing (2) by (1), we have

`(30)/(15) = (3Ae^(-3k))/(Ae^(-k)) \\2 = 3e^(-2k)\\(2)/(3) = e^(-2k)`

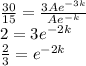
taking natural logarithm of both sides, we have

-2k = ㏑(2/3)

-2k = -0.4055

k = -0.4055/-2

k = 0.203

From (1)

`A = 15e^(k) \\A = 15e^(0.203) \\A = 15 X 1.225\\A = 18.36`

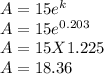
Substituting A and k into y, we have

`y = 18.36te^(-0.203t)`

The maximum value of y is obtained when dy/dt = 0

dy/dt = y(1/t - k) = 0

y(1/t - k) = 0

Since y ≠ 0, (1/t - k) = 0.

So, 1/t = k

t = 1/k

So, the maximum value of y is obtained when t = 1/k = 1/0.203 = 4.93 hours

`y = 18.36(1/0.203)e^(-0.203t)\\y = (18.36)/(0.203)e^(-0.203X1/0.203)\\y = 90.44e^(-1)\\y = 90.44 X 0.3679\\y = 33.27 g`

So the maximum amount of red kryptonite present is 33.27 g after 4.93 hours.