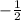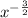Question

What is the derivative of1/root x​

Answer 1

`-(1)/(2)`
`x^{-(3)/(2)}`

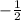
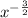
Step-by-step explanation:

Task: To find the derivative of 1/rootx

Rewrite: To find the derivative of the function 1 /
`√(x)`

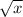
To find the derivative, follow these steps:

(i) Rewrite the function as

=>
`(1)/(√(x) )`

Remember that
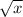`√(x)` can be written as
`x^{(1)/(2) }`

=>
`\frac{1}{x^{(1)/(2) } }`

=>
`x^{-(1)/(2) }`

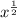
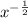
(ii) Multiply the coefficient of
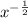`x^{-(1)/(2) }` by the power of
`x^{-(1)/(2) }`

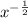
Coefficient of
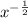`x^{-(1)/(2) }` = 1

Power of
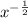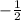
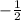`x^{-(1)/(2) }` =
`-(1)/(2)`

=> (
`-(1)/(2)` x 1)
`x^{-(1)/(2) }`

=> (
`-(1)/(2)` )
`x^{-(1)/(2) }`

=>
`-(1)/(2)`
`x^{-(1)/(2) }`

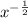
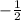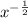
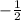
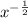
(iii) Subtract 1 from the power of
`x^{-(1)/(2) }`

=>
`-(1)/(2)`
`x^{(-(1)/(2) - 1 )}`

=>
`-(1)/(2)`
`x^{-(3)/(2)}`

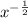
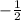
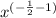
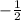
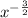
Therefore, the derivative of 1/root x is
`-(1)/(2)`
`x^{-(3)/(2)}`