Question

C

Which points are reflections of each other across the y-axis?
8
6
1 2
24
-9 -6 -4 -2
-2
2
ܘܠܐ
6 8 x
-4
-6
-8
(-7 -3) and (7-3)
(-5 4) and (5.-4)
(1, -8) and (18)

Answer 1

`(a)\ (-7,-3)\ \&\ (7,-3)`

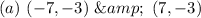
Explanation:

Given

Options a, b and c

Required

Points that are reflections across y-axis

The rule of reflection across the y-axis is:

`(x,y) \to (-x,y)`

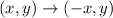
Testing the given options:

`(a)\ (-7,-3)\ \&\ (7,-3)`

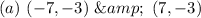
Rule:
`(x,y) \to (-x,y)`

`(-7,-3) \to (-(-7),-3)`

`(-7,-3) \to (7,-3)`

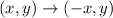
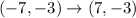
This is true, because the actual reflection equals the given reflection

`(b)\ (-5,4)\ \&\ (5,-4)`

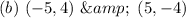
Rule:
`(x,y) \to (-x,y)`

`(-5,4) \to (-(-5),4)`

`(-5,4) \to (5,4)`

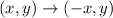
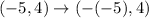
This is not true, because the actual reflection does not equal to the given reflection

`(c)\ (1,-8)\ \&\ (1,8)`

Rule:
`(x,y) \to (-x,y)`

`(1,-8) \to (-1,-8)`

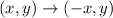
This is not true, because the actual reflection does not equal to the given reflection

Hence

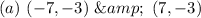`(a)\ (-7,-3)\ \&\ (7,-3)` is a reflection of each other across y axis